Bitcoin Private And Public Key Generator
17.12.2020 admin
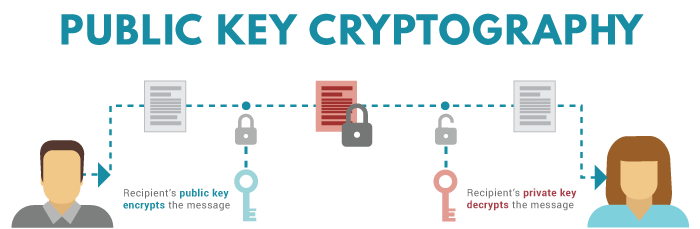
Private and Public Keys
- Bitcoin Public Key Generator
- Bitcoin Public And Private Key Generator
- Bitcoin Private Key Generator Blockchain
- Bitcoin Private Key List
- Bitcoin Public Key And Private Key Generator
- Public Key Example
Bitcoin Generation Calculator; StartMiner is Bitcoin miner with fully automatic process. Disclosure: bitcoin generation calculator was ist elektronischer handel. Private Key: xxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxx Public Key: 2Uc973e5adf5867512dd7a21638810bd53422 Private Key (WIF Format): xxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxx I have my concerns about the correctness of the generated keys, as well as for the private key in WIF format. Feb 12, 2015 As seen in our guides to elliptic curve cryptography and how to create a Bitcoin Private key – a public key is in fact just coordinates on the Bitcoin curve calculated through multiplying the generator point by the private key number. X coordinate= 7a633d546e723c3f2f2a227b6d393b35303d38. Private and Public Keys A bitcoin wallet contains a collection of key pairs, each consisting of a private key and a public key. The private key (k) is a number, usually picked at random. From the private key, we use elliptic curve multiplication, a one-way cryptographic function, to generate a public key (K). Oct 30, 2017 Get your private key from 24 word recovery phrase without Ledger Nano S (Update with Transactions) - Duration: 12:50. Blockchain and APPs 8,292 views. Of course bitcoin private key generator, btc bruteforce, bitcrack supports Windows and MAC Os Platforms. And some of the latest mobile platforms. For questions, support or error report please contact us.
A bitcoin wallet contains a collection of key pairs, each consisting of a private key and a public key. The private key (k) is a number, usually picked at random. From the private key, we use elliptic curve multiplication, a one-way cryptographic function, to generate a public key (K). From the public key (K), we use a one-way cryptographic hash function to generate a bitcoin address (A). In this section we will start with generating the private key, look at the elliptic curve math that is used to turn that into a public key, and finally, generate a bitcoin address from the public key.
Private Keys
A private key is simply a number, picked at random. Ownership and control over the private key is the root of user control over all funds associated with the corresponding bitcoin address. The private key is used to create signatures that are required to spend bitcoins by proving ownership of funds used in a transaction. The private key must remain secret at all times, as revealing it to a third party is equivalent to giving them control over the bitcoins secured by that key.
The private key must also be backed up and protected from accidental loss, since if lost it cannot be recovered and the funds secured by it are forever lost too.
Generating a private key from a random number
The first and most important step in generating keys is to find a secure source of entropy, or randomness. Creating a bitcoin key is essentially the same as “Pick a number between 1 and 2^256“. The exact method you use to pick that number does not matter as long as it Is not predictable or repeatable.
Bitcoin software uses the underlying operating system’s random number generators to produce 256 bits of entropy (randomness). Usually, the OS random number generator is initialized by a human source of randomness, which is why you may be asked to wiggle your mouse around for a few seconds. For the truly paranoid, nothing beats dice, pencil and paper.
How to see all keys
All Bitcoin private keys is simply an integer between number 1 and 115792089237316195423570985008687907852837564279074904382605163141518161494337 or HEX: from 1 to 0xfffffffffffffffffffffffffffffffebaaedce6af48a03bbfd25e8cd0364141. The integer range of valid private keys is governed by the secp256k1 ECDSA standard used by Bitcoin.
We just generate a range of these integers in sequence, divide into pages and show on each page. We can't store it and we have not saved database, because it should be biggest base on the world.
You can find Private key in WIF (Wallet Import/Export Format) and compressed key. Bitcoin addresses in compressed/ uncompressed formats, SegWit (P2SH-P2WPKH) and native Segwit (P2WPKH) addesses start bc1, Pay to script hash (P2SH) starting with 3; legacy Bitcoin Cash addresses and new format.
Bitcoin Public Key Generator
In the previous article, we looked at different methods to generate a private key. Whatever method you choose, you’ll end up with 32 bytes of data. Here’s the one that we got at the end of that article:
60cf347dbc59d31c1358c8e5cf5e45b822ab85b79cb32a9f3d98184779a9efc2
We’ll use this private key throughout the article to derive both a public key and the address for the Bitcoin wallet.
What we want to do is to apply a series of conversions to the private key to get a public key and then a wallet address. Most of these conversions are called hash functions. These hash functions are one-way conversions that can’t be reversed. We won’t go to the mechanics of the functions themselves — there are plenty of great articles that cover that. Instead, we will look at how using these functions in the correct order can lead you to the Bitcoin wallet address that you can use.
Elliptic Curve Cryptography
The first thing we need to do is to apply the ECDSA or Elliptic Curve Digital Signature Algorithm to our private key. An elliptic curve is a curve defined by the equation y² = x³ + ax + b with a chosen a and b. There is a whole family of such curves that are widely known and used. Bitcoin uses the secp256k1 curve. If you want to learn more about Elliptic Curve Cryptography, I’ll refer you to this article.
By applying the ECDSA to the private key, we get a 64-byte integer. /powerpoint-2013-product-key-generator.html. This consists of two 32-byte integers that represent the X and Y of the point on the elliptic curve, concatenated together.
For our example, we got: 1e7bcc70c72770dbb72fea022e8a6d07f814d2ebe4de9ae3f7af75bf706902a7b73ff919898c836396a6b0c96812c3213b99372050853bd1678da0ead14487d7.
In Python, it would look like this:
Note: as you can see from the code, before I used a method from the ecdsa module, I decoded the private key using codecs. This is relevant more to the Python and less to the algorithm itself, but I will explain what are we doing here to remove possible confusion.
In Python, there are at least two classes that can keep the private and public keys: “str” and “bytes”. The first is a string and the second is a byte array. Cryptographic methods in Python work with a “bytes” class, taking it as input and returning it as the result.
Now, there’s a little catch: a string, say, 4f3c does not equal the byte array 4f3c, it equals the byte array with two elements, O<. And that’s what codecs.decode method does: it converts a string into a byte array. That will be the same for all cryptographic manipulations that we’ll do in this article.
Public key
Once we’re done with the ECDSA, all we need to do is to add the bytes 0x04 at the start of our public key. The result is a Bitcoin full public key, which is equal to: 041e7bcc70c72770dbb72fea022e8a6d07f814d2ebe4de9ae3f7af75bf706902a7b73ff919898c836396a6b0c96812c3213b99372050853bd1678da0ead14487d7 for us.
Compressed public key
But we can do better. As you might remember, the public key is some point (X, Y) on the curve. We know the curve, and for each X there are only two Ys that define the point which lies on that curve. So why keep Y? Instead, let’s keep X and the sign of Y. Later, we can derive Y from that if needed.
The specifics are as follows: we take X from the ECDSA public key. Now, we add the 0x02 if the last byte of Y is even, and the byte 0x03 if the last byte is odd.
In our case, the last byte is odd, so we add 0x03 to get the compressed public key: 031e7bcc70c72770dbb72fea022e8a6d07f814d2ebe4de9ae3f7af75bf706902a7. This key contains the same information, but it’s almost twice as short as the uncompressed key. Cool!
Previously, wallet software used long, full versions of public keys, but now most of it has switched to compressed keys.
Encrypting the public key
From now on, we need to make a wallet address. Whatever method of getting the public key you choose, it goes through the same procedure. Obviously, the addresses will differ. In this article, we will go with the compressed version.
What we need to do here is to apply SHA-256 to the public key, and then apply RIPEMD-160 to the result. The order is important.
SHA-256 and RIPEMD-160 are two hash functions, and again, we won’t go into the details of how they work. What matters is that now we have 160-bit integer, which will be used for further modifications. Let’s call that an encrypted public key. For our example, the encrypted public key is 453233600a96384bb8d73d400984117ac84d7e8b.
Here’s how we encrypt the public key in Python:
Adding the network byte
The Bitcoin has two networks, main and test. The main network is the network that all people use to transfer the coins. The test network was created — you guessed it — to test new features and software.
We want to generate an address to use it on the mainnet, so we need to add 0x00 bytes to the encrypted public key. The result is 00453233600a96384bb8d73d400984117ac84d7e8b. For the testnet, that would be 0x6f bytes.
Checksum
Now we need to calculate the checksum of our mainnet key. The idea of checksum is to make sure that the data (in our case, the key) wasn’t corrupted during transmission. The wallet software should look at the checksum and mark the address as invalid if the checksum mismatches.
To calculate the checksum of the key, we need to apply SHA-256 twice and then take first 4 bytes of the result. For our example, the double SHA-256 is 512f43c48517a75e58a7ec4c554ecd1a8f9603c891b46325006abf39c5c6b995 and therefore the checksum is 512f43c4 (note that 4 bytes is 8 hex digits). Endnote x7 product key generator mac.
Bitcoin Public And Private Key Generator
The code to calculate an address checksum is the following:
Getting the address
Finally, to make an address, we just concatenate the mainnet key and the checksum. That makes it 00453233600a96384bb8d73d400984117ac84d7e8b512f43c4 for our example.
That’s it! That’s the wallet address for the private key at the start of the article.
But you may notice that something is off. You’ve probably seen a handful of Bitcoin addresses and they didn’t look like that. Well, the reason is that they are encoded with Base58. It’s a little bit odd.
Here’s the algorithm to convert a hex address to the Base58 address:
What we get is 17JsmEygbbEUEpvt4PFtYaTeSqfb9ki1F1, a compressed Bitcoin wallet address.
Conclusion
The wallet key generation process can be split into four steps:
Bitcoin Private Key Generator Blockchain
- creating a public key with ECDSA
- encrypting the key with SHA-256 and RIPEMD-160
- calculating the checksum with double SHA-256
- encoding the key with Base58.
Bitcoin Private Key List
Depending on the form of public key (full or compressed), we get different addresses, but both are perfectly valid.
Here’s the full algorithm for the uncompressed public key:
Bitcoin Public Key And Private Key Generator

If you want to play with the code, I published it to the Github repository.
I am making a course on cryptocurrencies here on freeCodeCamp News. The first part is a detailed description of the blockchain.
Public Key Example
I also post random thoughts about crypto on Twitter, so you might want to check it out.